Fun with Picture Grids: Teaching Square Root
Order a set at: http://www.hand2mind.com/catalog/product?deptId=&prodId=020272&q=picture+gridsPart VI: Teaching Square Root with the Picture Grid Manipulatives
In a previous article, Henry Goodman took you on a journey that proved it was not only possible to teach students mathematics, but possible to help them understand how mathematics works. Through the use of both rods and grids, Henry was able to help students visualize the mathematics problems that had previously remained a mystery.
The good news is that Henry Goodman is back. The other news which may be either good or bad is that he is determined to teach square roots. Ugh! The students recoil in horror! The teacher, desperate and without any means of support, wrings her hands and wonders, “What can I do? Oh, what can I do?” At this point in the script, our hero, Henry Goodman, enters. He brandishes his trusty tools. He is back, and he has brought Picture Grids with him. Once again, everyone is safe. The enemy – square roots – can and will be overcome. Everyone breathes a deep and steady sigh of relief!
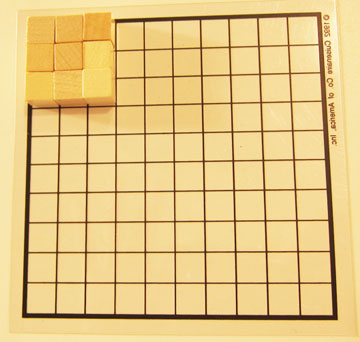
“All right now,” Henry says, “I think we’re ready for squares and square roots.” He removes the units from the Cuisenaire rods to use in combination with the grids. “Take out the hundredths clear grid and place it in front of you.” (It is sometimes best to use a white piece of paper under the grid. Colors will show up better and make the problem easier to visualize.) “Now, in the corner of the grid, create a square with nine units.” He gives the groups sets of blocks and waits until they finish.
Then he holds up the blocks.
Illustration: Clear 100s, with 9 unit cubes from Cuisenaire Rods, in the upper left-hand corner
“A square root is equal to one side of the square. Rotate the grid and count the number of blocks on each side. What’s your answer?” The students all say, “Three.” They understand that all the sides are equal because the figure is a square.
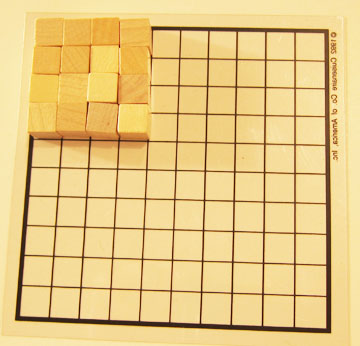
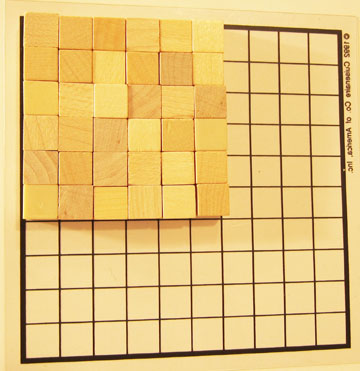
Next, Henry has the class try the same exercise with sixteen. They come up with the square root of four. Henry continues to give them more problems. They use twenty-five units for a square root of five, and thirty-six units for a square root of six.
Illustrations: 16 cubes
Illustrations: 25 cubes
For middle school students, the following exercise is a bit more challenging and sophisticated. First, ask students to figure out the square root of ten. Tell them to build the largest square they can. The result will be a three by three square that equals nine with one leftover.
Answer: 9 plus one leftover
Now ask the students to pretend for a moment. What can we do to this leftover unit so that it will conform to our concept of a square? Some students suggest that the unit be cut into thin slabs and placed around the sides of the existing square. Other students realize that it’s only necessary to place the slabs on one length and one width. They place three on the bottom and three slabs on the side.
Another student determines that while this is not exactly the answer, it is quite close. Henry asks the students to draw it, so they can visualize it. One student says, “We’re missing the corner piece.”
The students say, “So, we need to cut the leftover unit into seven pieces to create a square.”
Henry encourages the students to determine the size of the square that follows the one already created. One student says, “We’ve already created a three by three, so then the next size up is a four by four.” Henry says, “How many units will we need to add to the outside of the three by three in order to create a four by four?” A student says, “Seven” Henry nods and continues. “Therefore,” he says, “the one Cuisenaire unit would have to be cut into seven ‘very thin’ slabs. The corner slab would then have to be cut more than two times in order to fit into the corner.”
Henry tells the students, “The approximation of the square root of ten would be three whole squares plus what?” One student answers, “Approximately one-seventh?” Henry asks the students to use a calculator to compare their results of the square root of ten. They see that the answer is about three and one-seventh. Now students can make larger squares and determine the square roots. Henry gives one group the number 19:
19 = One four by four + the three left over from the next outside square of nine.
So.. .the answer is 4 3/9.
Once the students are familiar with the material, they will become familiar with the square roots and be able to automatically calculate the roots in their heads.
At this point, students are encouraged to create their own scenarios with the grids to practice. With repetition, they get the hang of it. Then, Henry asks them to write one of their scenarios along with the diagrams in their Pic-Jour Math journal. Not until Henry is convinced that the pictures/diagrams are strongly linked to the numbers, does Henry move them away from the manipulatives and just use numbers in his teaching practices.
Part VII: Games
Students can also play games with the Fraction Grids. On a large sheet of paper, create three areas that students can use for their grids.
Students can place the original picture grid on the left side, keep the middle free, and use the right side for the answer. Students can play My Operation or Operation. Each student can attempt to solve the mystery of his partner’s solution to the problem by using the grids. Students can copy the problem, draw the results, and place a box around the answer. These sheets can be turned in to the teacher for an outcome-based assessment. For example, begin with one-third. The end could display a large box divided into twelve equal parts with one area shaded darker to indicate one-twelfth. What operation was used? What fraction is missing?
One answer could be determined by placing the quarters grid over the thirds grid until it is evident that one-third is multiplied by one-fourth.
Or, a student could take the clear grids and lay each one over the 1/3rd to determine twelfths. He would discover that ¼ of 1/3rd = 1/12th
Shrinking the Square is another game that students can play. Some students prefer to play with partners or against an opponent. The purpose of the game is to begin with a whole square and to shrink it. Take a blank die and write one-half, one-third, one quarter, one-sixth, and one-twelfth on its sides. Take a whole grid and place it in front of you. Roll the die and subtract the amount that is rolled. As the game continues, the students will need to keep rolling until the fraction needed is rolled.
In a previous article, we saw that the grids are an invaluable tool for teaching addition, subtraction, multiplication, or decimals. Now it is clear that Picture Grids help students visualize squares and square roots so that understanding is as much a given as the right answer.
The suggested games are representative of the many ways that teachers can simplify the concepts essential for understanding fractions. Because the grids can be maneuvered, students can actually see the sections as they are divided and learn the reasons for their answers. When students are encouraged to develop a visual understanding of how numbers are used, numbers are no longer a mystifying body of incomprehensible symbols.
(Together with a visual understanding of numbers comes a new reality that is born of comprehension.)
Speech: A Math Manipulative Association of Mathematics Teachers of New York State, Buffalo, NY© 1993
A Math Manipulative to Teach Fractions and Decimals, © 1993 Speech by Andi Stix, Ed.D.Association of Mathematics Teachers of New York State, Buffalo, NY,ERIC: Eric Resources Information Center #ED408158 (c) 1992 Picture Grids designed by Andi StixProduced by ETA/Cuisenaire Company of America.Andi Stix is an educational consultant & coach who specializes in differentiation, interactive learning, writing across the curriculum, classroom coaching, and gifted education. For further information on her specialties or social media, please email her on the Contact page.
References
Schubert, B. (1987). Mathematics journals: Fourth grade. In Toby Fulwiler (Ed.), The Journal Book. (348-358). Portsmouth, NH Boynton/Cook Publishers.
Stix, Andi N. (1992).The Development and Field Testing of a Multi-Modal Method for Teaching Mathematical Concepts to Preservice Teachers by Utilizing Pictorial Journal Writing. Ph.D. diss. Columbia University Teachers College. Ann Arbor, MI: U.M.I. Dissertation Information Service, Pub. #92-18719.
Stix, Andi N. (1994). Pictorial journal writing in mathematics. Arithmetic Teacher, 42:1, 264-269.